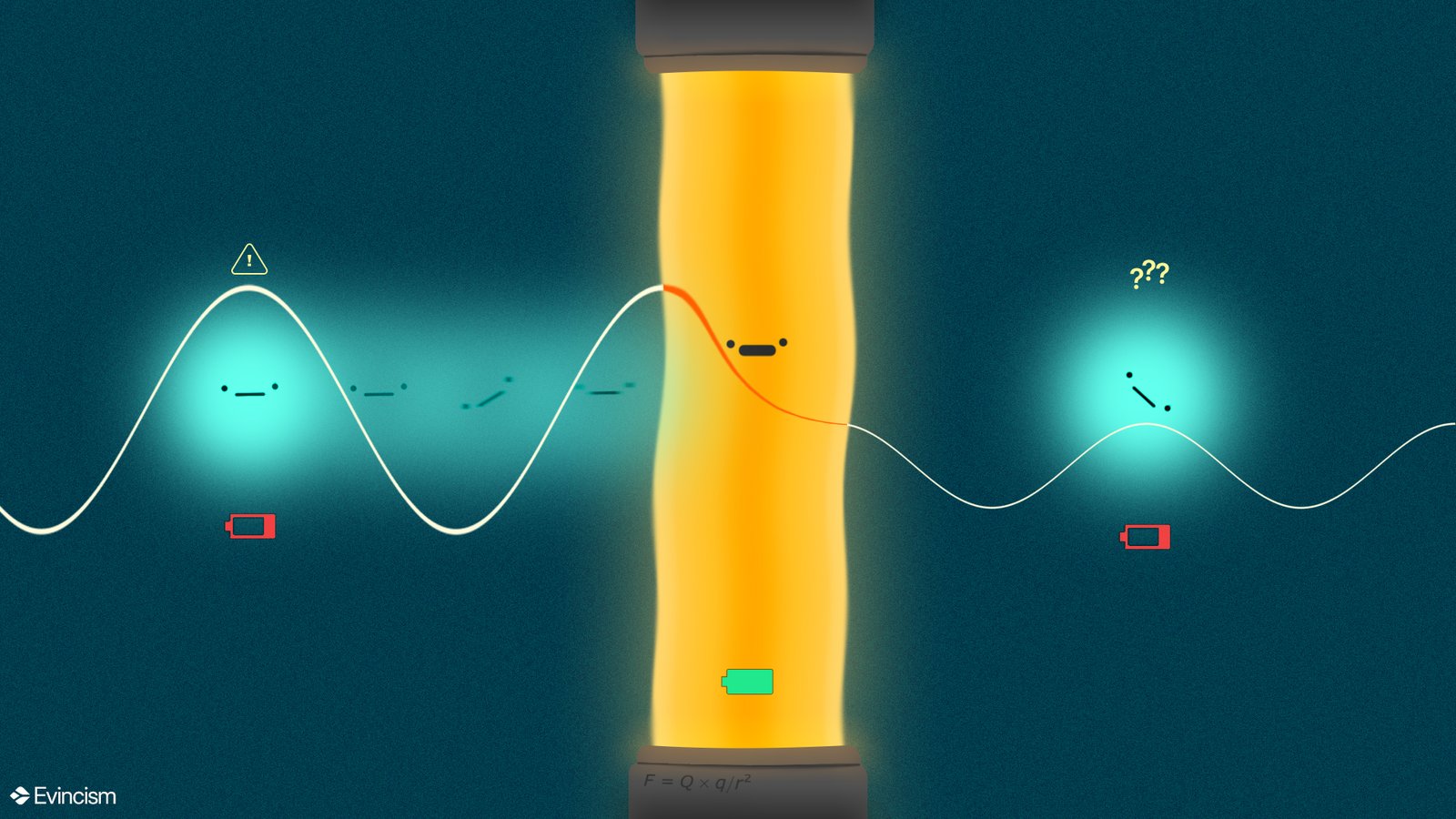
Suppose you are running towards a wall, presuming you’re not a very bulky person but just an average human supposedly you’ll hit your head, and the rest is pain.
That’s at least in classical physics but considering quantum physics, there is another possibility.
In Quantum Physics, you may run into a wall and find yourself on the other side. Well, that’s indeed weird but this is how Quantum tunneling works.
But wait you pity-classically-restricted-human, the human and the wall example above is used as a metaphor, so have some fun while you can.
Quantum tunneling only works for very tiny things such as an atom, electron, proton et cetera. (Why? the reasons will get clear as you read further)
In this article, we will explore this phenomenon by hitting up our intellectual heads onto the solutions of the Schrodinger equation in varying potential fields. I am joking(I have to).
Understanding how and why quantum tunneling happens will require the basics of quantum mechanics.
We will try going deeper into what picture of the quantum world the wave-particle duality paints and how wave function describes the cloud-like model of atoms, which allows them to be spooky and do spookier things.
Quantum Mechanics in a Nutshell
This will be a quick overview of some quantum mechanical concepts, to get you familiar with how things work the way they do in the quantum world.
What’s a Quantum object?
In general, quantum mechanics is only applicable to systems that exhibit quantum behavior, such as particles that exhibit wave-particle duality and are subject to the uncertainty principle.
These systems are known as quantum objects, and they are typically much smaller than objects that can be described using classical mechanics.
The Wave-Particle Duality
In the 19th century, scientists believed that light is a wave, later, Max Planck showed that light travels, is emitted, and absorbed in the form of discrete energy packets called quanta.
Einstein used Planck’s idea further and showed that light has a dual nature i.e. it can behave like a particle as well as a wave.
A Physicist Louis De Broglie, argued that not just light but all matter showed this wave-particle duality.
Physicists conducted the double-slit experiment (the same experiment was conducted by Thomas Young to test the wave nature of light, earlier) using an electron beam this time.
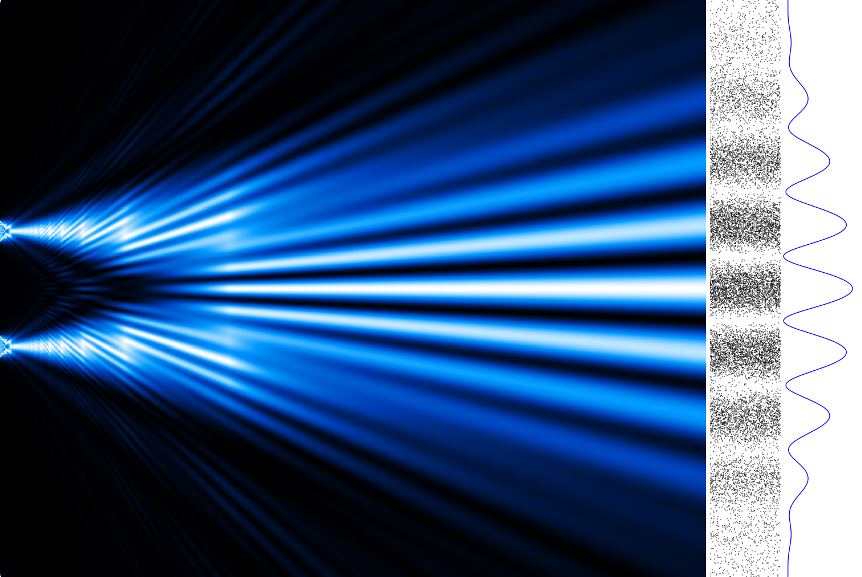
They found results similar to that as shown by light and this meant electrons too behave like a wave.
Since it was true for an electron, it must be also true for atoms, molecules, a ball, you, and even our Earth.
We don’t normally experience this wave nature ourselves because at the macroscopic scale, their wavelength is just so small that it’s very hard to detect them.
So, De Broglie was right.
The Heisenberg’s Uncertainty Principle
After wave-particle duality, a Theoretical Physicist Werner Heisenberg figured out something even more astonishing.
He suggested if the wavelength of a quantum object such as an electron is significant and detectable, we will not be able to observe the position and momentum of the quantum object precisely at the same time.

If we obtain a precise measurement for the momentum of an electron, then we would mess up with the position of the electron.
This implies you cannot simultaneously predict a precise value for the position or momentum of a quantum object, with both of those parameters there will be some uncertainty associated.
You can better understand it this way, when we make an observation it happens by interacting with the system with photons.
As the photon collides with the electron, due to the collision, it will introduce uncertainty in both the position and the momentum of the electron.
This created a problem for Bohr’s model of the Atom as it was based on the principle that electrons revolve around the nucleus at a ‘particular’ velocity and that makes it rather predictable which is not the case in reality.

Since electrons just like photons, also reflect a wave-particle behavior, we can no longer say that electrons revolve around the nucleus all neat and clean. It was required to redefine the atomic structure.
Enters Erwin Schrödinger with the Schrödinger-Equation
A few years later, Erwin Schrödinger came up with a solution.
He said that probability is a huge factor in figuring out how atoms and molecules behave in reality.
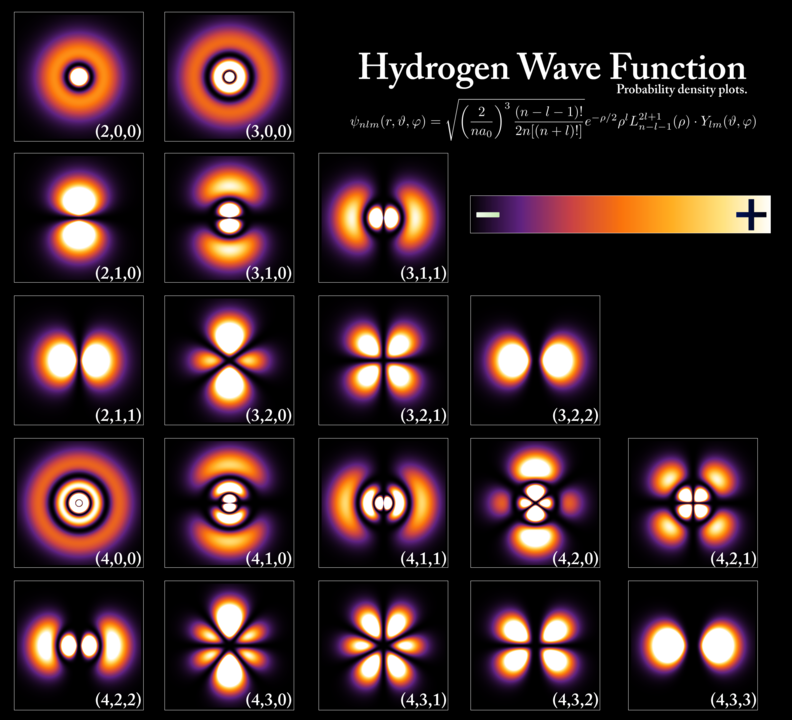
It led to the establishment of the currently ruling quantum mechanical model of an atom, also known as the cloud model.
Here, the probability of finding an electron around the nucleus is considered and we use orbitals to show the probability of finding electrons in different regions around the nucleus of an atom.
Schrodinger introduced an equation that is now called ‘The Schrödinger Equation‘, which helps us find the probability of finding a quantum object in a certain region of space.
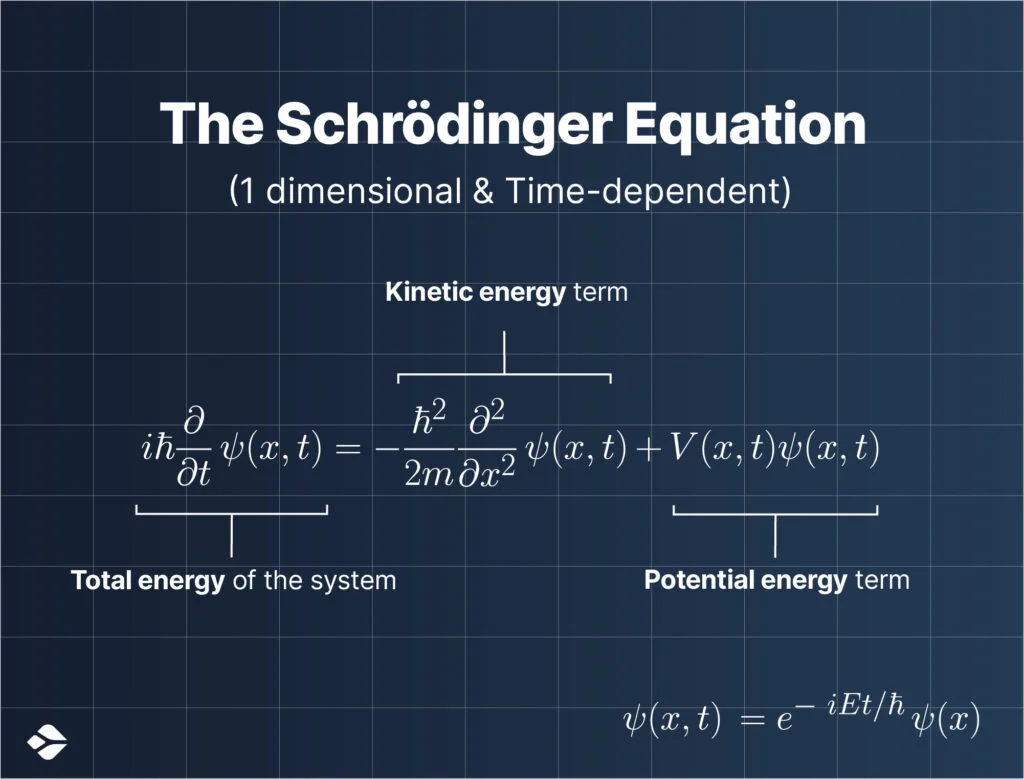
Above is the Schrödinger Wave Equation, the one in the image is a time-dependent, 1-dimensional special case equation (which makes things a bit relatively easier to understand).
It considers that the given quantum object traverses only in 1 dimension, the x-axis.
The equation as a whole is based on the conservation of energy (as labeled in the image).
Here, $\:\psi(x,t)\:$ is a wave function, it assigns a complex number to each point $\:x\:$ at each time $\:t\:$; $\:m\:$ is the mass of the particle, and $\:V(x,t)\:$ is the potential that represents the environment in which the particle exists.
The constant $\:i\:$ is the imaginary unit, and $\:\hbar\:$ is the reduced Planck constant, which has units of energy multiplied by time.
Here, the equation also describes the evolution of something called the wave function, of a quantum object over time.
As you can see in the image, the wave function $\:\psi(x,t)\:$ is differentiated with respect to time (on the left-hand side of the equation).
Stay put!
What is a Wave function ($\psi$)?

The above is what a wave function graphically looks like.
A wave function mathematically describes the quantum state of an isolated quantum system such as an atom, a molecule, or a subatomic particle depending upon your concern – with the help of probability.
What is a Quantum State
A quantum state refers to the probability distribution of each possible physical measurement (position, momentum, energy et cetera) in a quantum system.
What is a Probability Distribution
Probability distribution tells us about the likelihood of certain possible outcomes for an event.
For instance, let’s say a bag has 5 apples and 2 oranges, using common sense, if you were to pick a fruit from the bag randomly, it’s more likely that you will pick up an apple.
A probability distribution for the above case will represent a higher chance for the apple to be picked up.
In the wave function graph you saw above, we can see that the curve dips into negative values as well.
This doesn’t mean that the probability of finding an electron is negative, although the wave function can have both positive and negative values.
We use Schrödinger’s Equation to solve for $\:\psi\:$, and then square the modulus of the wave function $\:|\psi|^2\:$, which is called the probability density function to filter out the imaginary part (if you noticed there’s the complex number $\:i\:$ in the equation) and ensures that the probability is always non-negative to get a proper probability distribution.
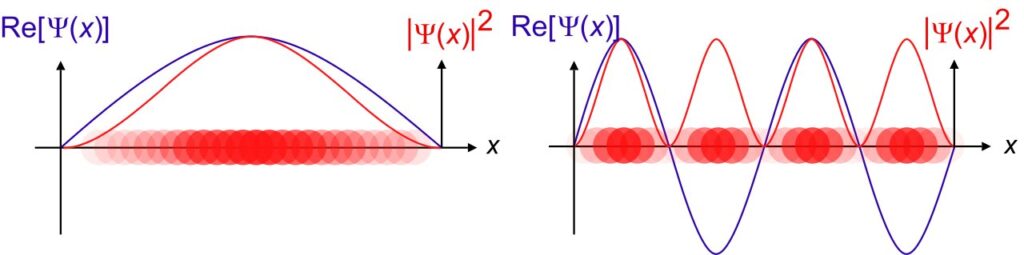
It generally gives the probability of finding an electron in a region of volume around a certain position or point.
For example, let’s put an electron inside a box, we don’t know the location of the electron inside the box.
In this case, the probability density function will tell us about the probability of finding an electron at different places inside the box.
Certain places will possess a higher probability while other places will have a lower chance for the electron to be in there.
You may actually say that the electron is at multiple places inside the box at the same time, as an electron’s wave function can be non-zero at multiple locations in space, however, this does not necessarily mean that the electron is indeed at multiple places at the same time.
The wave function describes the probability of finding the electron at a particular location, but it does not provide any information about the actual location of the electron.
There are also places where the probability of finding the electron is zero.
After we make an observation, we’ll find the cute innocent electron in one place, but also not necessarily determined by the probability density function (you may also find it at places where the probability density was zero after collision things go messy).
This idea of an electron behaving to be at different places at the same time is known as quantum superposition.
So basically, unless an observation is made, a quantum particle is said to exist in all of the possible states, simultaneously, however, we lack any information if it does practically exist like that simultaneously.
Meet the Wave-Packet
There is yet another mathematical tool that we use to describe a quantum object – a wave packet.
As you know we cannot simultaneously measure the position and momentum of a quantum object, to ease up things a bit more, we use a wave packet.

It is a partially localized wave of a quantum object, a compromise between the position and momentum variables.
This basically means that the uncertainty in the position and momentum is kept as much less as possible while satisfying the equation of the uncertainty principle.
So instead of a highly uncertain spread wave, we have a small envelope wave with relatively less uncertainty in the position and momentum variables.
We extensively use wave packets to denote quantum objects, and it is very helpful as there is less uncertainty in the physical properties.
Potential Barrier
Now that you know some quantum physics, there is another term you need to know about, it’s called a potential barrier.
In the quantum world, these barriers can be anything – a wall of charged atoms, another charged particle, just a resisting force, or just something that won’t allow the quantum object to go across unless it has enough energy to do so.
That’s all pretty much of the prerequisites (I know that wasn’t very quick), now let’s come get back to our topic.
Quantum Tunneling in Action
Technically, the entire concept of Quantum Tunneling is that a quantum object such as an electron surpasses a potential barrier, which supposedly in the eyes of Classical Physics mustn’t happen.
It is essentially because of the fact that electron doesn’t have enough energy to do so –
You’ll run into a wall and rather find yourself crying instead of getting teleported to the other side of the wall.
Retracing back to the metaphorical example, the wall is like a potential barrier for us in that case.
According to classical physics, a particle cannot possibly tunnel through the barrier given that it doesn’t have enough energy.
But now after the situation is not so ‘classical’, we know, that the actual picture of a particle is more like a probability cloud or wave, and being such probability clouds, particles become very spooky.
One way to understand quantum tunneling is through Heisenberg’s Uncertainty principle –
The uncertainty in the exact location of a particle, allows them to break the rules of classical physics and move through the barrier without having enough energy.
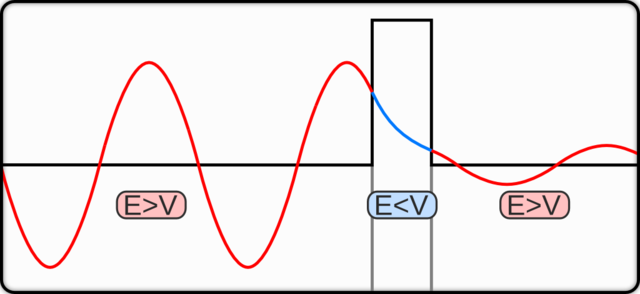
Notice in the above image, that the rectangular block at the mid-right is the potential barrier.
You can also see the probability wave of the particle which has less energy than what is required to surpass the barrier.
Technically speaking – Inside the barrier, the potential $\:V\:$ is greater than the particle’s energy $\:E\:$.
After the collision with the barrier, there is an exponential decrease in the probability amplitude of the quantum particle, also called a decay function.
Wave functions are complex-valued functions of the form $\psi (x)= Ne^{ikx}$, where $\:N\:$ is a constant and $e^{ikx}$ is a complex number with a magnitude of 1.
This complex number describes the phase of the wave function at a given point in space, and the value of $\:k\:$ determines the wave number of the wave function.
When a particle encounters a potential barrier, the probability amplitude of the wave function decreases exponentially inside the barrier, resulting in a reduced probability of finding the particle inside the barrier.
If the barrier is not wide enough, the wave function will still have some non-zero probability amplitude on the other side of the barrier, meaning there is a non-zero probability of finding the particle on the other side.
However, this probability will be smaller than it was before the particle encountered the barrier.
The energy of the particle remains unchanged in this process.

In the graphic above, you can see a wave packet of a particle incident on a potential barrier.
On the horizontal axis is the position and on the vertical axis, we have three parameters –
$\psi\:$ is the wave function, $\:V\:$ is the barrier energy, and $\:E\:$ is the mean energy of the wave packet.
A wave with a smaller amplitude emerges on the other side of the barrier with the same mean energy.
Hoof, I guess by now you’ve become a pro at this (kidding), so that’s pretty much it about the quantum tunneling mechanism.
Probability of Quantum Tunneling of a Particle
To make it easy for you to understand, let us move forward to one of the most important aspects of quantum tunneling – the tunneling probability i.e., the probability of a particle tunneling through a potential barrier.
For that, we’ll have to deal with the Schrödinger equation – $-\dfrac{\hslash^2}{2m} \, \dfrac{\mathrm{d}^2 \psi}{\mathrm{d} x^2} + V\psi = E\psi$
Now, let’s consider the case of rectangular potential, where we have three regions – Regions I, II, and III. In regions I and III, the potential is zero but for region II it is $V_0$ spanning from x = 0 to x = L.
So, the solution of $\psi$ would be different in these regions. Let the solutions of $\psi$ for these regions be $\psi_1$, $\psi_2$, and $\psi_3$ for regions I, II, and III respectively.
The Schrödinger equation can then be written as:
$\frac{d^2 \psi}{dx^2} + \frac{2m}{\hslash^2} (E-V) \psi = 0 $
But since the value of V is zero in regions I and III,
$\frac{d^2 \psi}{dx^2} + \frac{2m}{\hslash^2} (E) \psi = 0 $
Let us not dive into the various methods to solve a differential equation and directly jump to the solution that is,
$\psi_1 = A e^{ikx} \ + \ Be^{-ikx}$ and $ \psi_3 = Ee^{ikx} \ + \ Fe^{-ikx}$,
where $k = \frac{\sqrt{2mE}}{\hslash}$
If you’re left scratching your head in confusion, we suggest you stay with us, for it gets interesting ahead.
Now, suppose that the particle is approaching the barrier from the left. After colliding with the barrier, some part of it is reflected back while some of it is transmitted.
On observing the solution of $\psi_1$ (look at the equation above), we notice that it consists of two terms, out of which, the first term depicts a wave traveling towards the positive x direction while the second term depicts a wave traveling in the negative x direction.
So, $\psi_1$ represents both the incident and reflected wave function of the particle.
Similarly, the wave function of a transmitted particle would have had only one term as the particle would be moving through and away from the barrier i.e.,
$ \psi_3 = Ee^{ikx}$
Now, let’s come to the solution of the second region. Since we have a non-zero potential here, let’s rewrite the Schrödinger equation for simplicity.
$\frac{d^2 \psi}{dx^2} – a^2\psi = 0$
Where, $a = \frac{\sqrt{2m(V-E}}{\hslash}$
The solution to this equation would be,
$\psi_2 = Ce^{-ax} + De^{ax}$
Now, we have five unknowns – A, B, C, D, and E. These are arbitrary constants and we can find the relationship between them by applying the boundary conditions.
But what are those boundary conditions, or one can ask, what are boundary conditions in the first place?
Boundary conditions are like the borders of the mathematical realm, guiding how things behave at the edges or boundaries or borders of our mathematical premise. They specify values or relationships that the system must satisfy at its boundaries.
For a wave function in quantum mechanics, there are two common boundary conditions:
1. The wave function must be zero at the boundaries of a system. It means that the wave function completely vanishes at those points. In our case, those boundaries are at infinity as we are considering that a particle, coming from infinity, vanishes at infinity.
$\psi(\infty) = \psi(-\infty) = 0$
2. The wave function must be continuous everywhere. So, while passing through the potential barrier, the wave function must remain in continuity.
$\lim_{x\to 0^-} \psi(x) = \lim_{x\to 0^+} \psi(x) $
3. The wave function must be smooth everywhere. It means that there should not be any bending or breaking of the function in space. It can be ensured by considering the continuity of the derivative of the wave function everywhere.
$\lim_{x\to 0^-} \psi'(x) = \lim_{x\to 0^+} \psi'(x)$
By applying these boundary conditions, we can obtain the relationship between the arbitrary constants that we briefly discussed.
These arbitrary constants are important as they provide us with information about the intensity of the reflection or transmission of the wave function i.e. they act like weights or coefficients.
Or in other words, the ratio of the square of E and A would give us the probability of the particle being transmitted or tunneled through the potential barrier.
By directly jumping onto the result, we see that,
$T = \frac{|E|^2}{|A|^2} = \frac{16}{4+(\frac{a}{k})^2} e^{-2aL} $
The quantity in fraction varies much less with E and V than does the exponential term. So, it can be approximated to 1 for reasonable conditions. Hence we have,
$ T = e^{-2aL} $
Let’s take an example of an electron with energy 1eV incident on the potential barrier of energy 10eV and 0.5nm wide.
Putting all these values in the above equation, we get the value of the tunneling probability T = 1.1×10-7.
It means that if 9×106 electrons are incident on the barrier, only one will tunnel through the barrier.
The mathematics of probability and quantum mechanics are what decodes the phenomena of quantum tunneling, which has much more to it than just a particle (or a living being) going through a wall. Nonetheless, it is amusing.
Does Quantum Tunneling Violate the Conservation of Energy?
One of the most obvious questions that may arise is that if the particle with low energy tunnels through the potential barrier, does it mean that it gains energy from nowhere to tunnel through?
Well, quantum mechanics is not about energy but the probability of physical properties associated with a quantum object.
Here, we don’t know why certain phenomena happen, we just know that they happen.
Turns out, quantum mechanics seems to allow the violation of the law of conservation of energy implicitly, because of the uncertainty principle, but no not at all.
The uncertainty principle is a fundamental principle in quantum mechanics that describes the limitations of measurement, but it does not imply that the law of conservation of energy can be violated.
A system can make a transition to a state that violates conservation energy by an amount $\:\Delta E\:$ as long as it stays in that state for a time $\:\Delta t$ while satisfying the expression:
$\Delta E \cdot \Delta t\geq \frac{\hbar}{2}$
This energy-time principle states that a quantum state that lasts for a short period of time cannot have a definite energy[1].
Since the frequency of a state is inversely proportional to time and the frequency is linked to the state’s energy, the state must be observed for several cycles to accurately calculate the energy.
This is a form of Heisenberg’s uncertainty principle dealing with the trade-off between the energy measured and the period for which it’s been observed.
To conclude, we don’t know what an individual particle is going through, and hence there is always some uncertainty regarding its energy and a lot of other things.
Hence, there is always a small non-zero chance of finding the quantum object on the other side of the barrier.
Real-life Quantum Tunneling Applications & Examples
Quantum effects are more prevalent in nature than you think, they are happening all around you, including the device you are using to read this article.
They are the reason the Sun and other stars in the sky exist, which in turn is the reason we exist.
Quantum tunneling happens inside stars including the sun, it happens inside your devices, it happens even in plants during photosynthesis and even inside your DNA mechanisms. I am not kidding.
Quantum Tunneling in the Sun & Stars
Stars have to keep fusing atoms inside their cores to fight their own oppressing gravity.
So what’s the problem with nuclear fusion inside stars?
In nuclear fusion, two atoms come together to fuse and form a new atom of a different chemical element.
The electrostatic repulsion between the atoms hinders nuclear fusion because it’s basically like two proton balls coming close together.
This repulsion is called the coulomb barrier (a potential barrier) in the nuclear fusion process. So two atoms inside a star require enough kinetic energy to overcome the coulomb barrier.
The kinetic energy of atoms is related to the temperature of stars.
The temperature of the Sun’s core is about 106-7 kelvins or so (tens of million Kelvins), which is still not high enough to overcome the Coulomb barrier, which is as high as 109 kelvins or so, but thanks to quantum tunneling the nuclear fusion proceeds.
Quantum Tunneling in Semiconductors
The influence of quantum effects particularly tunneling on semiconductors is two-fold. Quantum tunneling can cause problems and is deemed an obstruction in various scenarios.
On the flip side, it also has been helping in revolutionizing the world’s computing abilities.
Leakage Current in Semiconductors Due to Quantum Effects
Although, in a circuit, we can manipulate a semiconductor to not allow any current to pass through.
However there is some small current that flows, and this leakage is more noticeable as you keep reducing the physical size of the semiconductor.
This is called the leakage current, which is because of the quantum tunneling effect.
In smaller semiconductors, the size of the energy barrier in its depletion region is not enough to restrict the electrons on either side of the energy barrier from tunneling.
Quantum Tunneling in Transistors
Transistors are semiconductors used in all modern devices, they are used to perform all kinds of binary logic and amplify electrical signals in electronic devices.
There is Moore’s Law that says the number of transistors in a chip has to double every year to aid in computing power.
Since the chip’s physical size can’t be increased, the transistor’s size has to be reduced every year.
And we just learned, that by reducing the size of the transistor which is a semiconductor, the quantum effects inside it will become prevalent and ruin its functioning.
Using Quantum Tunneling for Flash Memory Storage
You would be familiar with computer storage equipment like Micro SD cards, USB flash drives, and SSDs (Solid-state drives).
Unlike hard disk drives, these do not have any moving parts, so they last longer and have also contributed to revolutionizing computing.
Quantum Tunneling is used in these flash memory chips to store and manage information.
Quantum Tunneling in Tunnel Diodes
Tunnel diodes are used for high-speed switching and as microwave frequency amplifiers.
Quantum Tunneling is used to create an apparent “negative” differential resistance in Tunnel Diodes which allows them to be used as amplifiers, oscillators, and switching circuits.
Quantum Tunneling Scanning Tunneling Microscope
Scanning tunneling microscopes are used to capture a clear image of atomic surfaces.
Quantum tunneling is used in scanning tunneling microscopes to allow electrons to tunnel through the vacuum between the tip of the microscope and the sample surface.
This tunneling current is used to create a topographic map of the sample surface.
Quantum Tunneling in Radioactive Decay
Radioactive decay is a process in which an unstable nucleus spontaneously emits radiation in the form of alpha particles, beta particles, or gamma rays.
The emission of radiation is a result of the decay of the nucleus, which is an unstable configuration of protons and neutrons.
The decay of the nucleus is a random process, but it is governed by the laws of quantum mechanics.
The quantum tunneling effect allows particles to tunnel through barriers that would be impenetrable according to classical mechanics.
Quantum tunneling is responsible for the decay of the nucleus, as the particles that make up the nucleus can tunnel through the energy barrier that separates the initial and final states of the nucleus.
Can Humans Quantum Tunnel out through a wall?

Of course! If the wall is made of paper.
Well, the straight answer is NO (practically thinking).
There are a few clear reasons why quantum tunneling is not for us macroscopic beings –
For starters, it only works for the lighter particles, like the electron whose mass is way lower when compared to that of a human.
The solution of the wave function ‘Ψ‘ from the Schrödinger equation as mentioned above in the article, is inversely proportional to the object’s mass and hence quantum effects will vanish due to the higher mass of us humans.
Also, while talking about humans we’re talking about quintillions of atoms that we’re made up of.
Quantum effects are significant when relatively fewer particles are involved, but as the number of particles increases, it becomes exponentially harder to observe any quantum effect.
You could get a trillion people walking into walls, a trillion times every second since the beginning of the universe (13.8 billion years ago) – and the likelihood of one of them walking through the wall is still so small, it’s practically zero.’[2]
Jack Fraser, Physics Graduate, University of Oxford
Hence, you better open a door and ditch the running-into-the-wall idea.
Wrapping up the Tunneling Effect in Quantum Mechanics
In quantum tunneling, a quantum object, which is any tiny particle in the quantum world, surpasses an energy barrier even without having enough energy to do so.
Now it is just a matter of “how does it happen”, to understand, that there is no “why” that we know clearly due to the ambiguity in the quantum world.
In quantum mechanics, we represent quantum objects as probability clouds, which is basically a probability distribution chart giving us a probability of finding the quantum object in certain places of a region.
As this probability cloud of a quantum object encounters an energy barrier, a portion of the cloud slides through the barrier, making a way out to the other side due to which there exists a small probability of finding the quantum object on the other side of the barrier if it’s not wide enough.
Frequently Asked Questions
How wide should be the barrier to allow Quantum Tunneling
Tunneling usually occurs with barriers of thickness around 1-3 nm and smaller[3].
It also depends on the height and width of the potential barrier–if it’s too high (high energy) or too thick (physical width), tunneling won’t happen.
Factors that Affect Quantum Tunneling
The physical width of the energy barrier and the amount of energy of the barrier are the two factors that affect quantum tunneling.
Can particle Quantum Tunneling out of a Black Hole?
On the edges of Black Holes, quantum tunneling may be possible, however, in regions much closer to the singularity the barrier energy is too high to allow quantum tunneling.
Recommendations
Devesh Sharma, and Vikrant Singh, ‘Quantum Mechanics Explained: Mathematical Guide for Beginners‘, Evincism, 24 February 2023
Pranav Mahapatra, ‘Most Stars Shouldn’t Exist – Quantum Tunneling in the Sun‘, Evincism, 20 December 2022
References
- ‘7.3: The Heisenberg Uncertainty Principle‘, Libre Texts Physics, 1 November 2016, “a quantum state that exists for only a short time cannot have a definite energy”[↩]
- Jack Fraser, ‘What is the probability of a person walking through a wall based on quantum mechanics‘, Quora, 14 June 2016, “Or rather, it’s a number which isn’t zero, but is too small for any computer to even begin to calculate.”[↩]
- Rita G Lerner, and George L Trigg, ‘Encyclopedia of Physics‘, (2nd edition) New York: VCH., p.[1308], https://archive.org/details/encyclopediaofph00lern/page/1308/mode/2up[↩]