Quantum Mechanics Explained: Mathematical Guide for Beginners

Quantum Mechanics is one of those branches of physics that has taken the world by storm ever since its birth.

It is arguably the most popular field not just among professional physicists but also among armchair physicists (that’s a fancy little term you might want to google) and science enthusiasts.

Being something that we have all been hearing about since school, quantum mechanics is the most bizarre and complicated description of the universe, or so is its stereotype in the ordinary world.

Doing physics is like a game of chess, except the physicists are the spectators trying to guess what’s going on in the minds of the players (if you can guess who they are) by hypothesizing ideas and formulating abstract algorithms.

The ideas of quantum mechanics are usually (almost always) unintuitive, which is perhaps because it involves concepts that concern matter that is too tiny and not directly observable, which is also why the quantum world doesn’t follow what we intuitively understand from the directly observable world.

Nonetheless, if you ask quantum physicists about it, they would say that it is much easier for them as compared to other branches of physics (like classical mechanics). 

That is because quantum mechanics studies the simplest systems of the universe, such as a free elementary particle.

So is that it, or is there much more to why quantum mechanics is as hyped?

What does quantum mechanics tell us about the universe and how did it emerge and evolve to explain reality as we know it?

Let us raise all curtains, and dive deep into the immersive quantum realm to understand how and why quantum mechanics is one of the biggest (if not “the” biggest) revolutions in all of physics.

What is Quantum Mechanics?

You might have heard people define quantum mechanics as the branch of science that studies subatomic particles and their behavior at the smallest scales.

Although that is true by all means, there is more to quantum mechanics than just that.

Quantum mechanics is a theoretical framework that attempts to devise an elegant description of all processes in the universe by explaining the nature of reality at the most fundamental scale and how processes at this scale give rise to classical mechanics and other branches of physics. 

Quantum mechanics did not come from classical mechanics; instead, classical mechanics is an approximation of quantum mechanics in some sense.

That is indeed a very abstract (and mouthful) definition, but we want you to understand “What does quantum mechanics do?”, instead of just telling you “What is quantum mechanics?”. 

All things in this universe share an underlying fundamental reality and it is that fundamental reality that quantum mechanics tries to explain.

It is not only about subatomic particles and their superposition; it is about absolute truth.

Since this article touches on the elementary mathematics of quantum mechanics, we assume that you are familiar with some basic prerequisites like vectors, matrices, and differential equations (an equation involving derivatives of a quantity).

If not, there is nothing to worry about, with minimal rigor, you will never be left out of a conversation over quantum mechanics after reading this article.

Schrödinger Equation – Describing the Quantum World

Anyone curious about quantum mechanics by a good margin must have heard about the famous Schrödinger equation.

The Schrödinger equation or Schrödinger wave equation was developed by Austrian physicist Erwin Schrödinger in 1926, it is one of the most foundational equations in all of quantum mechanics.

Schrödinger wave equation describes the evolution of a quantum mechanical system over space and time (equation of motion in quantum mechanics), this can be expressed mathematically by the equation below:

$\frac{\partial}{\partial t}\Psi(\mathbf{r},t) = -iH\Psi(\mathbf{r},t)$

The above equation is the simplest known form of the Schrödinger equation that is known to mankind. 

With basic knowledge of high-school calculus, it can be understood that the above equation talks about the change of a quantity, which is represented by the Greek letter $\Psi$ (psi), with respect to time on the left-hand side.

The letter $\Psi$ is what represents our quantum system, which is the subject of our interest.

This change with respect to time equals the “imaginary” quantity (because it contains the imaginary number $i$ or iota) on the right-hand side, which is just $H$ multiplied by the same quantity as the one on the left-hand side 

The $H$ which stands for the ‘Hamiltonian operator’, is not a number but rather something that is represented by a matrix, which we will discuss later in this article.

The above equation was a reduced form of the Schrödinger wave equation. You might have seen the expanded equation alternatively.

The Schrödinger equation is based on the idea that a quantum system can be further described by something called a wave function.

A wave function can be roughly interpreted as a mathematical function that encodes all possible quantum states that the system can be in.

The Schrödinger wave equation describes how this wave function (and thus, the quantum system) changes over time, based on the characteristics of the quantum system like energy.

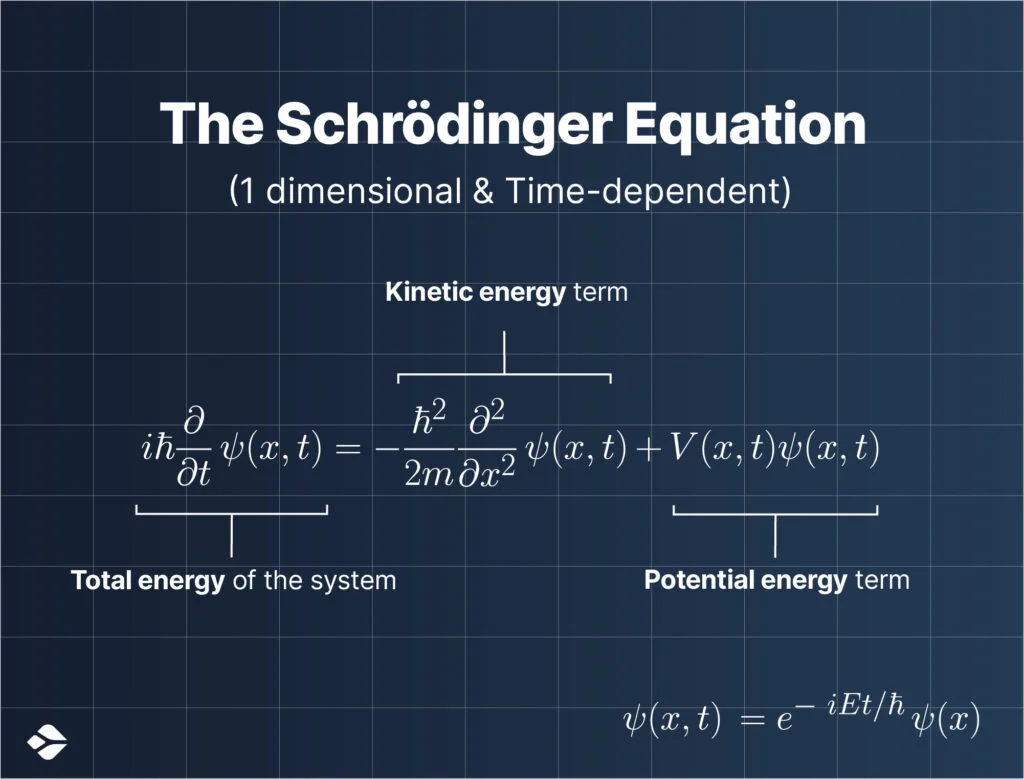
Now in the above image, this is what you would call the Schrödinger equation in its full glory.

It can also be reduced slightly:

$ih\frac{\partial}{\partial t}\Psi(\mathbf{r},t) = \frac{-\hbar^{2}}{2 m} \nabla^{2}\Psi(\mathbf{r},t)+V(\mathbf{r})\Psi(\mathbf{r},t)$

The Schrödinger equation is a (partial) differential equation, which means that it involves the rates of change of certain quantities. 

In the case of the Schrödinger equation, these quantities are the wave function and the energy of the quantum system. 

The left-hand side of the equation represents the total energy of the system, while the right-hand side of the equation consists of two terms that are kinetic energy and potential energy respectively. 

So, we can also say that the Schrödinger equation obeys and represents the law of conservation of energy. 

What’s $ih \frac{\partial}{\partial t}$ and $\frac{-\hbar^{2}}{2 m} \nabla^{2}$ out there and what do they mean? 

Those are called operators which extract information about the observable quantities of a quantum system (like its energy, momentum, position, etc).

Above all, the most important thing is to understand what $\Psi(r,t)$ means.

Of course, it is the wave function but there is much more to a wave function than just a wave-like function.

Wave Function $\Psi$ – Mediators of the Quantum World

The wave function is a mathematical function that describes the state of a quantum system.

As mentioned earlier, all quantities about the system that a scientist is interested in measuring can be extracted from the wave function by performing certain operations on it.

What separates quantum mechanics from classical mechanics is the probabilistic approach of quantum theory, all that we are interested in can be expressed as probabilities. 

Unlike a classical system that tells us where to find the particle after some time given its initial variables, quantum mechanics solves the probability of finding the position of the particle in a specific region or point of spacetime.

It is the wave function that provides information about the probability of finding a particle in a particle region, having a particular energy.

However, the wavefunction $\Psi$ alone does not give the probability of a system.

It is the squared modulus of the wavefunction $|\Psi|^2$ which represents the probability density of the system; something which we will talk about next.

Note that the notations $\Psi$ and $\psi$ can both be alternatively used to denote either the wave function of a single particle or a quantum system, it depends upon the author and the context^[1].

So, the wave function is not a physical wave, but rather a mathematical function that describes the behavior of a quantum mechanical system.

In general, the wave function is a complex function, meaning that it can be written as a combination of a real part and an imaginary part, i.e, of the form $A+iB$. 

The imaginary part ($iB$) is generally included in the wave function because it is a convenient mathematical tool for describing certain properties of quantum systems.

But as we know by our physical experience, the particle is localized in nature, meaning it will either be present at a point or will not be present at all. 

So, what’s the meaning of such a function which is spread out in space over a region?

How does it represent a particle in space?

This was a hard thing to explain, even for Erwin Schrödinger, and was answered by the German physicist Max Born^[2]. 

Statistical Interpretation of Wave Function – Born Rule

$\Psi$ has no physical meaning of its own but the square of its amplitude, $|\Psi|^2$ does. It represents the probability amplitude of the particle(s) in a system.

This interpretation of the wave function is popularly known as Born’s statistical interpretation or simply as the Born rule.

The Born rule is one of the key postulates of quantum mechanics and was first proposed by Max Born in 1926 as a possible interpretation of the wave function. 

Prior to Born’s interpretation, the wave function was understood to be a mathematical tool for describing the behavior of quantum systems, but there was no clear physical interpretation of the wave function^[3].

Note that $\Psi$ does not give a single value, it is a continuous function that gives a certain value at every point in space. 

It means that the particle can be located at a given point where there is a probability of its existence. 

When we say squared modulus of $\Psi$, we do not mean the product of $\Psi$ with itself; instead, it implies the product of $\Psi$ with its complex conjugate $\Psi^*$.

This, then, gives us the square of $\Psi$, expressed as $\Psi \cdot \Psi^*  = |\Psi|^2$.

Any quantity when squared in quantum mechanics generally means multiplying it with its complex conjugate or hermitian conjugate.

Fact: Complex conjugate of a quantity is given by inverting the sign of the imaginary term in the quantity. So, for instance, if $\Psi = A + iB$, then $\Psi^* = A –\: iB$. 

We do it to eliminate the imaginary part because the imaginary part doesn’t have any physical meaning for us in our real world. 

As you can see for yourself, by using the property of imaginary numbers that iota squared equals -1 ($i^2 = \sqrt{-1}^2 = -1$), we can form a real number by multiplying an imaginary quantity by its complex conjugate, as we did with $\Psi$.

This gave us a real-valued probability amplitude $|\Psi|^2$, which makes sense because probability (amplitude) cannot be imaginary; it has to be real.

Since $|\Psi|^2$ gives the probability distribution over the space, upon integration over all space, we get:

$\int_{-\infty}^{\infty}\Psi \cdot \Psi^* dv = 1$ where $dv$ is the differential volume element.

This implies that the total probability of finding the particle is one, as it should be i.e. it must exist at least somewhere in space. 

So, we don’t know the definite location of the particle but we do have an idea about the probability of that particle being located at a certain point in space.

But if a particle is located at a certain point in space, why do we need the probabilistic picture having a distributed probability over a region of space?

Particle In a Box – Collapse of the Wave Function

A wave picture of a particle is only plausible when it is not being observed. 

Suppose you want to locate the particle inside a box (this is a typical problem in quantum mechanics known as “particle in a box”). 

In classical physics, the probability distribution of the position of a particle inside the box would be uniform but when we assume the quantum effects, that’s not quite the case. 

The shape of the wave function resembles a gaussian distribution with zero probability at the ends of the box. 

This means that there are more chances of finding the particle in the center of the box than near the edges. 

But remember, we’re not “looking” at the particle right now; the lid of the box is closed and there is no way to observe the particle.

All we have done is explain the behavior of the particle with the best theoretical tool that we have – quantum mechanics.

Once we open the box, we will find the particle at a particular position i.e. once the observation is made, the distributed wave function (or the distributed probability) collapses to become localized (search Dirac delta function for a better mathematical understanding).

This is the point beyond which probability makes no sense and the system has now been set in a state where the particle is at a specific position.

Close the lid again, open it a second time and you will still find the particle in the same place.

It is like fixing a system in a particular configuration, this is what we mean by observing a quantum system.

Fact: Note that “observation” in quantum mechanics does not simply mean looking at a subatomic particle with bare eyes. Observation is but an interaction between a classical and a quantum system. This interaction disturbs the quantum system and prepares the state in a specific configuration, or eigenstate.

So, one of the most bizarre things about quantum mechanics is that the state of the particle remains indeterminate until or unless an observation occurs, which leads us to the idea of superposition.

Principle of Superposition – “My Existence is Debatable”

The statistical interpretation of wave function gives rise to a fundamental phenomenon in quantum mechanics known as superposition.

The principle of superposition states that a quantum system can simultaneously exist in multiple states at the same time. 

Superposition is a consequence of the wave nature of matter at the microscopic level.

Since the wave nature of matter diminishes at the macroscopic level, it isn’t significant in the classical approach. 

In the statistical interpretation, we learned that a quantum system doesn’t have a definite state but rather has a probability associated with every state it could be in.

So, a wave function can be represented as the linear combination of the wave function of every single state. 

If $f(x)$ and $g(x)$ are two functions, then their linear combination would be represented mathematically as $y(x) = a\cdot f(x) + b\cdot g(x)$ where a and b are constants, which tell us how much each wave function i.e. $f(x)$ or $g(x)$ contributes to making $y(x)$. 

That is the purpose of those constants; they act as “weights”.  

If for a given quantum system, the Schrödinger equation gives two solutions $\phi (x)$ and $\theta (x)$ each representing a particular state, then their linear combination $\Psi (x) = a\cdot (x) + b\cdot \theta(x)$ would then be the general solution. 

Here ‘a’ and ‘b’ are constants and if $y(x)^2$ when integrated gives the value $1$, then $a^2$ and $b^2$ would give the probability of the system being in the respective state (this is directly given by Born Rule).

Hence, the system is in a superposition of two states unless it is observed, and it is the observation that then fixes the system in one particular state.

Schrödinger’s Cat – The Famous Quantum Mechanical Paradox 

One of the most famous explanations for the principle of superposition is the thought experiment proposed by Erwin Schrödinger in 1935, commonly known as Schrödinger’s Cat. 

In this thought experiment, Schrödinger put a cat in a box that contains a radioactive isotope which has a 50% chance of emitting a radioactive particle, and a poisonous gas sealed in a glass tube. 

Inside the box, there is also a radioactivity detector, which would trigger a hammer upon the sealed glass tube of poisonous gas on detecting the radioactive decay. 

If the decay occurs, the hammer will strike the glass tube releasing the poison, and the cat would cease to exist.

Now the probabilistic approach of quantum mechanics says that the state of a system is undetermined and can be in multiple states at the same time until it is observed.

So if there is a probability of the radioactive element to both decay and not decay, until or unless we open the box, we would not know if the cat is dead or alive; in other words, we would not know if the decay has occurred or not. 

There’s a 50% chance of it being alive or dead. Since the state of the radioactive particle is indeterminate, the state of the cat is also indeterminate. 

So, we say that the cat (due to the radioactive material, which decides the fate of the cat) is in the superposition of two states – alive and dead. 

So as long as the cat is inside the box and we do not observe the cat, it is both alive and dead at the same time. 

This is the famous paradox that goes by the name of Schrödinger’s Cat.

Hilbert Space – “Complex” Space of Quantum Mechanics

The Hilbert space is an infinite-dimensional space of every possible solution for the Schrödinger equation. 

If you’ve never heard of Hilbert space, or even “space” (no, not the cosmological space; the mathematical one) in general, then it might sound a little weird. 

What does an infinite-dimensional space mean and how can solutions to the Schrödinger equation exist in it?

So let us first start with probably the most fundamental entity in abstract mathematics and quantum mechanics – vector spaces (of which, Hilbert Space is one case).

Vector Space, Basis, and Dimensions

A space in mathematics is a set of elements or data (which can be numbers, vectors, functions, or even sets) with some definite properties and structure. 

Any set of such mathematical data is called a space, defined by two properties – vector addition and scalar multiplication.

We can say that three vectors belong to a vector space if we add two vectors lying in the set (or space) and get a third vector as the resultant sum. This is what is called the property of vector addition. 

So, if $V$ is a vector space, then

$ u+v = w;\; \forall\ u,v,w\in V$

Here, we can say that vectors u, v, and w belong to the vector space $V$ because they obey vector addition.

Similarly, if we take any vector and multiply it by a constant number (scalars), the resultant vector (product) should belong to the vector space. This is the property of scalar multiplication.

Consider the following: 

 $ a.u= y;\; \forall\ u,y\in V\ and \ a\in \mathbb{R}$

Here, we can say that $a$ belongs to the class of real numbers. So, when we multiplied vector $u$, which belongs to the vector space $V$, by the constant $a$, we got $y$ as the resultant vector, which also belongs to $V$.

In superposition, we learned that any wave function can be represented as a linear combination of two or more wave functions. 

So clearly, it follows the property of vector addition and scalar multiplication with complex numbers.

$\Psi(x) = a.\phi(x) + b.\theta(x);\ \ \forall \ \Psi,\phi,\theta \in V \ and \ a,b\in\mathbb{C}$

In quantum mechanics, the vectors we encounter are functions, so the space in which these quantum mechanical functions lie is a function space.

Now that we have an idea about spaces, let us talk about the dimensions of that space.

The dimensions of a space are determined by the bases (singular basis) of a vector space.

The basis is a set of vectors inside a vector space such that every element or vector in that vector space can be constructed by all possible linear combinations of those basis vectors. 

A very special property of these basis vectors is that they are linearly independent of each other. 

So if you have 3 basis vectors, linear independence means that there is no possible way of adding two of them in any combination and getting the third vector.

Let’s consider the 3D space we live in. It can be constructed using three mutually perpendicular vectors which we usually denote by $x,y,z$ axes (for now, I am not considering ‘t’ for the time axis in spacetime). 

But remember, only those elements can form a basis that are linearly independent of each other meaning they can’t be constructed by the linear combinations of each other.

So, simply speaking, the dimension of any space is given by the number of its basis vectors.

For example, there are three basis elements (or basis vectors) in the space we live in (x, y, and z-axis), that’s why we consider our space to be three-dimensional. 

Complex Vector Space of Quantum Mechanics

We have already seen the Schrödinger equation above, which is but an equation of motion in quantum mechanics (like $F = ma$ in classical mechanics), describing the change in quantum state over time.

Now, the particular solutions to the Schrödinger equation can be infinite, based on the state of the quantum system. 

Hence, the general solution of the Schrödinger equation can be represented as the linear combination of these infinite solutions. 

$\Psi = \sum_{i}^{\infty} c_{i}\Psi_{i}$

Where $\Psi_{i}$ represents the basis vectors of the space of our solutions.

Note that we are summing over index ‘i’, so $\Psi_{i}$ means $\Psi_{1},\: \Psi_{2},\: \Psi_{3},\: \Psi_{4}…$ and so on, upto infinity.

So we see that our basis vectors run up to infinity because there are infinite possible solutions to the Schrödinger equation, and what we are essentially doing is summing over all these possible solutions.

This complex (meaning that it contains complex-valued data, like complex numbers and complex functions) space of solutions in quantum mechanics is what we call the Hilbert space, and owing to the infinite basis vectors, we say that our Hilbert space is infinite-dimensional.

Hilbert space, given its properties, is the only space that could satisfy the properties of a much-needed wave function space for doing quantum mechanics. 

Hence, we can say that wave functions (which are solutions to the Schrödinger equation) live in the Hilbert space. 

Linear Transformation – How Quantum Mechanics “Operates”

Linear transformation, also known as linear mapping, is a process of mapping (correlation) between two vector spaces. 

We can operate an element of a vector space into a whole different vector space. 

In other words, it is a way to travel from one vector space to another. But how is it done?

Such a transformation is carried out by using some functions or matrices in such a way that the property of vector addition and scalar multiplication is preserved. 

These transforming functions are what we call operators. These operators are expressed in the form of matrices (singular matrix).

Matrices are but an array of complex (or real, depending on the matrix) elements or numbers, in rows and columns, governed by the rules of operations of linear algebra.

In quantum mechanics, operators are very important as they execute the idea of linear transformation. 

Linear transformation helps us to extract information about the quantum system by transforming its wave function into another vector space such as position space and momentum space.

Before learning more about operators, let us learn about the famous “bra-ket” notation of quantum mechanics, given by one of the most revolutionary physicists in the field – Paul Dirac. 

“Bra-Ket” Notation – Dirac Notation 

In quantum mechanics, you might have seen weird brackets enclosing weird symbols like this:

$ \langle \Psi|\Psi\rangle $ . . . . . (1)

Those symbols, as you would’ve known, represent the wave functions enclosed in angular brackets separated by a vertical bar in middle. 

This is the bra-ket (fancy wordplay for “bracket”) notation which consists of two symbols – “bra” $ \langle \Psi|$ and “ket” $|\Psi\rangle $ (no one knows what happened to ‘c’). 

Let us understand what these “bras” and “kets” mean.

Remember how the state of a quantum system is represented as a vector in the Hilbert space?

A ket (also sometimes called a “ket vector”) is what represents our state vector.

On the other hand, a bra (no naughty) represents the corresponding dual vector, which is nothing but the result of a mapping of our ket vector into another space. 

So, if $\phi$ and $\Psi$ are two state vectors, then their inner product would be $ \langle \phi|\Psi\rangle $. 

An inner product is the quantum mechanical equivalent of a dot product denoting an overlap between one state and another (the more the two states are correlated, the more will be their inner product).

By definition, the inner product is an operation that maps two vectors (say $p$ and $q$) of an inner product space over a field (consider inner product space $V$ defined over field $F$) to a scalar value (real or complex, depending upon the inner product space) which is an element of the field $F$.

It is a highly abstract idea in linear algebra and abstract mathematics, which is extremely crucial to quantum mechanics.

Inner products in quantum mechanics are used as tools that describe probability amplitude.

Say you have two states namely $\Psi$ and $\phi$, and a system (say, a particle) that can be in these states.

Assuming the particle is in state $\Psi$, then an inner product between $\Psi$ and $\phi$ would describe the probability of the particle in state $\Psi$ to be found in state $\phi$.

Such an inner product can be simply expressed using the bra-ket notation; all you need is a bra and a ket:

$ \langle \phi|\Psi\rangle $

Bra-ket notation simplifies the calculations in quantum mechanics and represents the wave functions and operators in a more “intuitive” way.

$ \langle \Psi|\Psi\rangle $ would always give 1 meaning that the inner product of a state with itself equals 1. It says that the probability of state $\Psi$ being in the state $\Psi$ is always 1, obviously. 

Remember, $\Psi$ is the wave function of the system which is the linear combination of every possible state. 

So, $|\Psi\rangle$ can always be written as: 

$|\Psi\rangle = c_{1}|\Psi_{1}\rangle + c_{2}|\Psi_{2}\rangle + c_{3}|\Psi_{3}\rangle + ….+ c_{n}|\Psi_{n}\rangle$

Where $|\Psi_{i}\rangle$ represents particular state and $c_{i}$ is a scalar associated with it such that –

$c_{1}^{2} + c_{2}^{2} + c_{3}^{2} + . . . . . . + c_{n}^{2}  = 1$

If we write $ \langle \Psi|\Psi_{n}\rangle$, then it would give the probability of occurrence of the n^th state in the system. 

$ \langle \Psi|\Psi_{i}\rangle = c_{i}^{2}  $

Operators in Quantum Mechanics

Be it, classical mechanics or quantum mechanics, if we are given a system at hand, our goal at the end of the day is to measure its observable quantities.

This measurable information about the system includes its momentum, position, energy, angular momentum, etc. 

Such measurable information is extracted from the system by the mathematical machinery of operators.

The only way to do this is to “operate” the wave function with different operators for their corresponding observables (observable quantities). 

Operators are basically linear transformations in Hilbert spaces. On transformation, it yields new state vectors or just numbers.

Suppose we want to know the value of a physical observable ‘A’. Let its operator be $\hat{A}$ that can be applied on wave function $\Phi$ as:

 $\langle A\rangle = \langle \Psi|\hat{A}|\Psi\rangle$

Here, $\langle A\rangle $ represents the average value of the observable A. 

It means that if we take a large number of identical systems which are represented by the same wave function $\Psi$ and take the measurement of a physical quantity A, then the average value of all measurements would be $\langle A\rangle$.

These operators help us to study the physically observable quantities of the quantum system.

However, the observables themselves are not the actual measurable value that we get in our experiments. 

After all, operators are just matrices, whereas our obtained experimental values are numbers (see the difference?).

These operators (matrices) act on the state vectors (vectors), which then yield what we perceive as our measured value (a number).

This action, described above, is understood by a very special equation in linear algebra and quantum mechanics known as the eigenvalue equation, which will help us make more sense of operators and linear transformations.

By now, you must have understood how each mathematical idea in quantum mechanics is so deeply interlinked with the others, all describing the same reality with perfect coordination.

This is why we say that the very heart of quantum mechanics (or any other field in physics, in general) is its subtle mathematics.

With the eigenvalue equation, the basic mathematics of quantum mechanics comes full circle.

Eigenvalue Equation – Eigenvectors & Eigenvalues

A big chunk of quantum mechanics stands on the shoulders of the eigenvalue equation.

The eigenvalue equation is not a fancy name for a special equation, like the Schrödinger or the Dirac equation. 

It is rather a general linear algebraic equation that describes the linear transformation (which we have talked about earlier in this article) and the action of operators in the Hilbert space.

Even the Schrödinger equation in quantum mechanics is an eigenvalue equation.

The eigenvalue equation is made up of three things: eigenvalues, eigenvectors, and linear operators. 

It is necessary to understand the representations in linear algebra to understand quantum mechanics. 

We know that in linear algebra, state vectors (or ket vectors, or kets) are just vectors in the Hilbert space, whereas operators are represented by matrices. 

This is how to make sense of this:

Operators are there to act on a certain system, with certain values of observables and give back the exact same system but with different values of observables (as systems undergo change).

This process, which can be accomplished in linear algebra by matrices and vectors, is represented by the eigenvalue equation.

If you are familiar with matrix multiplication, you must know that a matrix can act (by ‘act’, we generally mean ‘multiply’) on a vector to give a different vector.

That is why we say that operators execute linear transformation (you can call them “linear transformers” if you like).

So when a matrix acts (multiplies) on a vector (imagine an arrow of a particular length in a cartesian space), we get a different vector (the same arrow but now of a different length and pointing in a different direction).

But what we want in quantum mechanics is that if changes are occurring on a system (again, a vector of a particular length pointing in a particular direction), we should get the exact same system but with changed values (the same arrow pointing in the same direction but with changed length).

This is where the eigenvalue equation comes in. 

The arrow that we talked of can be thought of as an eigenvector (its direction should not change, meaning that the vector i.e. the system does not change), whereas its length can be thought of as the eigenvalue of that eigenvector i.e. the actual observable quantity of that system (just like length is a measurable quantity of the arrow).

This means that any matrix, which is operated on a certain vector, gives back the same vector multiplied by some scalar quantity (meaning that the vector has expanded or shrunk).

The vector is known as the eigenvector whereas the scalar quantity (representing its expansion or shrinking) is the eigenvalue of the eigenvector.

To sum up, an eigenvector can be thought of as a vector whose “directional properties” don’t change under a transformation. 

The eigenvalue equation is written in the following form:

$ A \hat{x} = \lambda \hat{x}$

Where ‘A’ is the transformation matrix, $\hat{x}$ is a vector, and ‘λ’ is its eigenvalue. 

The equation means that matrix ‘A’ acts on the vector and yields the value ‘λ’ associated with the vector.

In quantum mechanics, operators are represented in form of matrices and state vectors in the form of vectors. So, the eigenvalue of a system (state vector) gives us the value of the observable quantity that we are interested in. 

Since the state of a quantum system is denoted by vectors that participate in the eigenvalue equation as eigenvectors, these eigenvectors (or state vectors) are also known as eigenstates or eigenfunctions.

So if a momentum operator denoted by ‘P’ acts on a state vector and yields the eigenvalue ‘p’, it means that ‘p’ (eigenvalue) is the momentum of the system.

Note that an operator can have multiple eigenstates i.e. an entire spectrum of eigenstates on which the operator can act.

Eigenvalue equations help us to obtain those possible eigenstates for a particular operator. 

For example, the determinate state vector of total energy is given by the eigenstates of the Hamiltonian (the operator corresponding to the energy of the system). 

$ \hat{H} \Psi = E\Psi$

This is nothing but the time-independent Schrödinger equation, which is but an eigenvalue equation (as we mentioned earlier). 

So, we can solve the Schrödinger equation by solving for its eigenvectors, and its eigenvalues would give us the total energy of the system. 

Stationary States – Eigenstates of the Hamiltonian

In the past few sections, we have touched down on the heart of quantum mechanics – its mathematics.

If you jumped directly to this section, you are insisted to go through the above sections, and if you have come this far after them all, you are more than capable of understanding a handful of basic applications and ideas in quantum mechanics. 

Let us now throw more light on the eigenstates of the Schrödinger equation, and why are they assigned a special name.

The Schrödinger equation can only be solved as an eigenvalue problem if it is time-independent. 

In the time-dependent Schrödinger equation, the energy operator is in the form of a first-order time derivative of the wave function i.e. it describes the time evolution of the system.

$ih\frac{\partial}{\partial t}\Psi(\mathbf{r},t) = \frac{-\hbar^{2}}{2 m} \nabla^{2}\Psi(\mathbf{r},t)+V(\mathbf{r})\Psi(\mathbf{r},t)$

In other words, energy in quantum mechanics can be understood by how a system evolves in time. 

But for the time-independent system, ‘E’ does not represent the time evolution of the system, but rather the energy eigenvalue. 

$\widehat{H}\Psi = \widehat{E}\Psi$

The states obtained from the time-independent Schrödinger equation are known as stationary states. 

In other words, the stationary states are the eigenstates of the Hamiltonian operator (or total energy operator). 

These are the states that the Hamiltonian can act upon to yield an energy eigenvalue, which is the true measure of the energy of the system.

Such stationary states can be represented as follows:

$\Psi (x,t) = \Psi (x) f(t)$

Where $\Psi(x)$ is the function of x (position) alone while f(t) is a function of time alone. 

This representation is in the form of a special method to solve differential equations in physics known as the separation of variables. 

If a function is made up of two or more independent variables (like $\Psi(x,t)$ being a function of both position and time), then it can be represented as the product of two independent functions each made up of one variable ($\Psi(x)$ being a function of position only, and f(t) being a function of time only).

The function ‘f(t)’ can be solved by putting the above equation in a time-dependent Schrödinger equation, and you’ll find:

$f(x) = e^{-iEt/\hbar}$

Note that the time independence of the Schrödinger equation does not mean that the wave function doesn’t change (or evolve) with time. 

It means that the probability distribution remains the same (or stationary, thus the name) throughout time.  

$|\Psi|^2 =(\Psi e^{-iEt/\hbar} ). (\Psi e^{-iEt/\hbar})^*$

$ = |\Psi|^2$

Hence, a stationary state does evolve in time, but its properties and observables do not change with time. Such states are states of definite total energy.

Copenhagen Interpretation of Quantum Mechanics

We’ve discovered a lot of mathematical formulations now.

So, our next question must be, how does all of it manifest itself in our physical reality? 

Physicists asked themselves the same question and ended up with different interpretations of quantum mechanics. 

Some popular interpretations in quantum mechanics are the Copenhagen interpretation, many-worlds interpretation, Quantum Darwinism, etc.

Out of these, the Copenhagen Interpretation is one of the most influential interpretations to date. To understand it, let’s put some light on our little particle-in-a-box case. 

When we open the box to find the particle at a particular position, we may ask the following question: Was the particle always there at that particular position, even before the measurement? 

We cannot say for sure, but one can think about it in three different ways.

One might argue that the particle was always at the same place both before and after making the observation. 

This might make sense at first but if it turns out to be true, quantum mechanics will come to its knees. 

So, even though quantum mechanics might be correct, it gives an incomplete description of reality. 

Some physicists believed that the particle was nowhere before the observation was made. The act of measurement forced it to take that particular position. 

Then again, why did the particle “choose” that very position and not the other ones? 

It leaves us in an orthodox position and this is where the Copenhagen Interpretation comes into play. Neils Bohr was a major supporter of the Copenhagen interpretation. 

At last, comes the agnostic case, according to which, an agnostic would refuse to answer that question. 

You may think it’s a silly question, but it is not quite so. For an agnostic, there is no point in asking about the position of the particle without making any observation. 

Talking about the position of the particle even before looking at it does not make much sense (why do we even need to know the position before actually observing the system). 

We might also guess the position, but the only way to prove our guess right is to make an observation (or measurement).

To stand behind this mystery as realists of their time, physicists Albert Einstein, Boris Podolsky, and Nathan Rosen proposed a paradox called the Einstein–Podolsky–Rosen (EPR) Paradox. 

It was solved by John Stewart Bell in 1964 when he proposed his version of the EPR Paradox. 

Surprisingly, Bell’s version proved that if the realist point of view was correct, then quantum mechanics will not only be incomplete but also incorrect. 

Nonetheless, quantum mechanics has undoubtedly explained some of the most fundamental phenomena in nature, including those that couldn’t be explained by classical physics.

So, for obvious reasons, we cannot afford to adopt the realist view (or any other view for that matter) at the cost of quantum mechanics, which to date is one of the most revolutionary creations of mankind.

Recommendations

Pranav Mahapatra, ‘The Quantum Tunneling Effect – How Does it Work?‘, Evincism

Devesh Sharma, and Vikrant Singh, ‘History of the Standard Model in Particle Physics‘, Evincism

References

1. Physics Stack Exchange, ‘In Quantum Mechanics is there any difference between $\Psi$ and $\psi$‘, “No, it is just a matter of convention, which heavily depends on the context.”, https://physics.stackexchange.com/questions/373993/in-quantum-mechanics-is-there-any-difference-between-psi-and-psi[↩]
2. Shan Gao, ‘Meaning of the Wave Function’, Schrödinger originally regarded the wave function as a description of real physical wave. But this view met serious objections and was soon replaced by Born’s probability interpretation (Born 1926), which becomes the standard interpretation of the wave function today, https://arxiv.org/ftp/arxiv/papers/1001/1001.5085.pdf[↩]
3. Wikipedia, ‘Schrödinger Equation’, “The Schrödinger equation provides a way to calculate the wave function of a system and how it changes dynamically in time. However, the Schrödinger equation does not directly say what, exactly, the wave function is.”, https://en.wikipedia.org/wiki/Schr%C3%B6dinger_equation[↩]